Solar Power

The earth receives more energy from the Sun in just one hour than the world’s population uses in a whole year.

The total solar energy flux intercepted by the earth on any particular day is 4.2 X 10¹⁸ Watthours or 1.5 X 10²² Joules (or 6.26 X 10²⁰ Joules per hour ). This is equivalent to burning 360 billion tons of oil ( toe ) per day or 15 Billion toe per hour.

In fact the world’s total energy consumption of all forms in the year 2000 was only 4.24 X 10²⁰ Joules. In year 2005 it was 10,537 Mtoe (Source BP Statistical Review of World Energy 2006)

 

Solar Radiation

Sunlight comes in many colours, combining
low-energy infrared photons (1.1 eV) with high-energy ultraviolet
photons (3.5 eV) and all the visible-light photons between.

The graph below shows the spectrum of the
solar energy impinging on a plane, directly facing the sun, outside the
Earth’s atmosphere at the Earth’s mean distance from the Sun. The area
under the curve represents the total energy in the spectrum. Known as
the “Solar Constant” G₀, it is equal to 1367 Watts per square metre (W/m²).

The radiant energy falling within the visible
spectrum is about 43% of the total with about 52% in the infra red
region and 5% in the ultra violet region.

 

The graph below shows the energy at sea level.

Direct energy is the energy received directly from the sun.

Global energy includes energy diffused, scattered or reflected from clouds and energy re-radiated by the earth itself.

Energy received at sea level is about 1kW/m² at noon near the equator

 

Irradiance and Insolation

Total solar irradiance is
defined as the the amount of radiant energy emitted by the Sun over all
wavelengths, not just visible light, falling each second on a 1 square
metre perpendicular plane outside Earth’s atmosphere at a given distance
from the Sun. It is roughly constant, fluctuating by only a few parts
per thousand from day to day.

On the outer surface of the Earth’s atmosphere the irradiance is known as the solar constant and is equal to about 1367 Watts per square meter.

The amount of solar energy that actually
passes through the atmosphere and strikes a given area on the Earth over
a specific time varies with latitude and with the seasons as well as
the weather and is known as the insolation (incident solar radiation).

When he Sun is directly overhead the
insolation, that is the incident energy arriving on a surface on the
ground perpendicular to the Sun’s rays, is typically 1000 Watts per
square metre. This is due to the absorption of the Sun’s energy by the
Earth’s atmosphere which dissipates about 25% to 30% of the radiant
energy.

Insolation increases with altitude

The terms “irradiance” and “insolation” are often used interchangeably to mean the same thing.

 

Available Solar Energy

Since the Earth’s cross sectional area is 127,400,000 km², the total Sun’s power it intercepted by the Earth is 1.740×10¹⁷ Watts but as it rotates, no energy is received during the night and the
Sun’s energy is distributed across the Earth’s entire surface area so
that the average insolation is only one quarter of the solar constant or
about 342 Watts per square meter. Taking into account the seasonal and
climatic conditions the actual power reaching the ground generally
averages less than 200 Watts per square meter. Thus the average power
intercepted at any time by the earth’s surface is around 127.4 X 10⁶ X 10⁶ X 200 = 25.4 X 10¹⁵ Watts or 25,400 TeraWatts.

 

Integrating this power over the whole year the total solar energy received by the earth will be:

25,400 TW X 24 X 365 = 222,504,000 TeraWatthours (TWh)

 

To put this into perspective, the total
annual electrical energy (not the total energy) consumed in the world
from all sources in 2004 was 16,600 TWh. Thus the available solar energy
is over 13,000 times the world’s consumption. The solar energy must of
course be converted into electrical energy, but even with a low
conversion efficiency of only 8% the available energy will be 17,800,000
TWh or over a thousand times the consumption. Using the same low
conversion efficiency, the entire world’s electricity demand could be
supplied from a solar panel of 118,000 km². Theoretically this could be provided by six solar plants of 20,000 km²or
141 km per side, one plant in each of the hot, barren continental
deserts in Australia, China, the Middle East, Northern Africa, South
America and the USA or one large solar plant covering 1% of the Sahara
desert.

 

Unfortunately the Sun’s bounty can only be
harvested during daylight hours and some energy must be stored for use
during the hours of darkness and the requirement to distribute the
energy over great distances to where it is needed make this proposition
impractical. The example merely serves to illustrate the abundance of
the sun’s energy.

What is practical however is to build
smaller, more efficient solar power plants to serve the demands of local
communities using free solar energy when it is available in conjunction
with other other energy sources or some local energy storage where
possible. Despite this, less than 0.1% of the world’s primary energy
demand is supplied by solar energy.

 

Equivalent Hours of Full Sun (EHS)

Because of the variation in the intensity of the Sun’s radiation during the day and also the variations in the length of the day it is difficult to make comparisons of the Sun’s energy falling upon the Earth at different locations. The graph opposite shows an example in which the insolation reaches 1000 W/m2 at noon when the sun is at its highest point in the sky. An insolation of 1000 W/m2 is known as the “Full Sun”. Most of the time the incident energy is below this value because it depends on the angle of incidence of the Sun’s rays with the ground, increasing during the day from a very low value at dawn as the Sun rises to a peak at noon and falling again as the Sun sets. (See Angle of Incidence below). Similarly the insolation will be reduced as higher latitudes due to the effect of air mass – (See below). The graph also shows that, in this case, the total received energy over the 10 hours of daylight will be 3.5 kWh. If the insolation had been constant at 1000 W/m2 the same amount of energy would have been received in 3.5 hours. The Equivalent Hours of Full Sun is a measure of average insolation at different locations. In this case the EHS is 3.5 hours. The available solar energy and thus the Equivalent Hours of Full Sun (EHS) also depend on the atmospheric conditions of cloud cover and pollution. See Available Energy – Practical Systems below.   The concept of EHS is useful for comparing the potential of solar energy systems when installed at different geographic locations.

 

Capturing Solar Energy

Solar energy can be captured in two forms, either as heat or as electrical energy.

• Thermal Systems

Thermal systems capture the Sun’s heat energy (infra red radiation) in some form of solar collector and use it to mostly to provide hot water or for space heating, but the
heat can also used to generate electricity by heating the working fluid
in heat engine which in turn drives a generator.

• Photovoltaic Systems

Photovoltaic systems capture the sun’s
higher frequency radiation (visible and ultra violet) in an array of
semiconductor, photovoltaic cells which convert the radiant energy
directly into electricity.

 

The actual solar energy or insolation
reaching a solar collector or array depends on its position on the
Earth, its orientation and it also varies continuously with time as well
as weather conditions.

The amount of energy captured is directly proportional to the area of the Sun’s energy front intercepted by the collector.

 

Some Geometry

The orientation of the solar collector or the
photovoltaic array with respect to the position of the Sun is a major
determinant in the efficiency of the solar power system.

 

• Inclined Planes

Angle of Incidence The amount of energy impinging on a collector or array is directly proportional to the area of the radiation wave-front it intercepts. For optimum energy capture the collector must be perpendicular to the Sun’s rays when the angle of incidence is 90°. For a flat plate on the ground this occurs only when the Sun is directly overhead. Unfortunately unless you live in the tropics this will never be the case and solar arrays must be tilted towards the Sun to receive the maximum insolation.   When the incident energy is not perpendicular to the collector, the angle of incidence is (90° – Θ) and the effective area of the collector is A.cosΘ where A is the area of the collector and Θ is the deviation from perpendicular of the radiation.

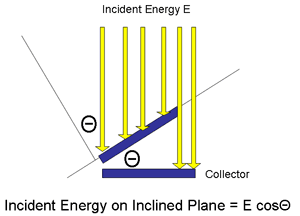
In the diagram above, the Air Mass corresponds to the factor (1/cosΦ)

Air Mass The Air Mass is a dimensionless quantity defined as the ratio between the actual path length of the solar radiation through the atmosphere and the vertical path length through the atmosphere at sea level. If the Sun’s radiation is not perpendicular to the Earth, the transit path through the Earth’s atmosphere will be longer and hence the energy absorbed on the way to the collector or array will be greater. The effect of the longer route through the atmosphere is to increase the energy absorption (or lost energy) by a factor of 1/cosΦ where Φ is the deviation from perpendicular of the radiation, also called the zenith angle. Thus in the polar regions as Φ approaches 90 degrees (cosΦ>0) the insolation is very low, even if the collector is pointed directly at the Sun, due to the longer path through the atmosphere.

 

• Altitude

Insolation increases with altitude since the
radiation passes through less air mass hence the energy absorption by
the atmosphere is less.

 

Some Astronomy

To calculate how solar insolation varies with
time and with the position of the collector on the Earth’s surface we
need to know a little astronomy.

 

Though the Earth moves around the Sun, for
the purposes of calculating the energy intercepted by our collectors it
is often convenient to assume that the Earth is stationary and the Sun
moves relative to the earth in much the same way as the ancients did
before Copernicus pointed out their error. Assuming the Earth does not
rotate, the apparent trajectory of the Sun follows a two-dimensional
plane in the sky called the ecliptic.

 

• Position

The position of the Sun in the sky relative to an observer on Earth is defined by its altitude angle α (solar elevation angle) and its azimuth angle Ψ.

 



• The Earth’s Orbit

The Earth orbits the Sun with one revolution per year in an
elliptical orbit with the Sun at one of the foci of the ellipse. The
orbit’s two foci are very close together however so that the orbit is
almost circular, the distance to the Sun from the perihelion, the point
in its orbit closest to the Sun, being only about 3% less than its
distance from the aphelion, its furthest distance.

 

Because the orbit is almost circular, the effect of the orbit
on solar irradiance remains essentially constant throughout the year as
the Earth orbits the Sun. The actual energy received at any distance
from the Sun is determined by the inverse square law. Thus a 3% change
in distance gives rise to a 6% change in the irradiance.

 

• The Earth’s Rotation

The Earth’s rotation of once per day defines our day and
night. As the Earth rotates the insolation at any point on its surface
rises to a maximum at mid day and falls to zero during the night as the
Earth presents a different face towards the Sun. For maximum efficiency
the orientation of the collector should follow the Sun as it passes
overhead from East to West.

 

• Latitude

A solar collector or array placed on the ground will only
receive the maximum insolation when the Sun is directly overhead.
Because the Earth is roughly spherical, the angle between the plane of
the Earth’s surface and the incident solar radiation will gradually
increase from 90 degrees as we move away from the equator to the upper
and lower latitudes by an angle Θ equal to the latitude of the observer. At this point the altitude angle α of the Sun will be (90 – Θ)
degrees. Because of the increased inclination of the Earth’s surface
the insolation received by a collector placed on the surface will
gradually decrease.

 

This drawback can be overcome by inclining the collector so
that it is perpendicular to the Sun’s rays. The amount of elevation from
the horizontal, the tilt angle, should be equal to the latitude angle Θ of the location of the collector.

 

For maximum effect the axis of the inclination should be
perpendicular to the polar axis. That is, in the Northern hemisphere the
direction of the collector should point due South.

Note that the polar axis is not the same as the compass
bearing because the magnetic poles do not necessarily line up exactly
with the geometric poles.
The angle between the magnetic and geographical meridians at any
place is called the magnetic declination or variation and can be as
much as 20 degrees or more. It is expressed in degrees east or west to
indicate the direction of magnetic north from true north.

 

Unfortunately the Sun does not appear to follow a constant
path in the Earth’s equatorial plane. It appears to move North in the
Summer and South in the Winter. In fact the Sun is stationary and the
effect is due to the tilt of the Earth’s axis of rotation.

 

• The Earth’s Tilt

The Earth’s rotational axis is tipped over about 23.45
degrees from the plane of its orbit. This tilt is essentially constant,
maintained in that direction due to the gyroscopic action of the earth’s
rotation, and always points in the same direction relative to the
stars, so that the North Pole points towards the star Polaris, the North
Star. Over very long time periods however, measured in thousands of
years, the direction of Earth’s axis slowly changes due to gyroscopic
precession.

 

 

The fixed orientation in space of the Earth’s axis as it
orbits the Sun determines the length of the day and creates the world’s
seasons. At the summer solstice, the longest day,. the northern half of
the Earth is pointing towards the Sun creating summer in the Northern
hemisphere. The winter solstice, the shortest day in the Northern
hemisphere occurs when the Earth has travelled 180 degrees around its
orbit and the Northern hemisphere is pointing away from the Sun.

From the Earth it appears that the Earth’s rotational axis is
rocking backwards and forwards. The apparent tilt of the Earth’s axis
corresponds to the angular position of the Sun at its highest point in
the sky with respect to an observation point on the plane of the equator
and is called the solar declination δ (Not to be confused with magnetic variation, also called the declination).

The vernal (spring) and autumnal equinoxes, in March and
September when the day and night are the same length, occur when the
Earth is mid way between the solstices. Then the plane of the tilt is
perpendicular to the direction of the Sun from the Earth so that the
insolation is the same on both hemispheres.

 

As a result of the Earth’s tilt, the intensity of the
insolation varies during the year giving rise to the seasons. This is
not because tilt causes a point on the Earth’s surface to move closer to
or further from the Sun. The change in distance is negligible. It is
because of three factors:

• The Earth’s apparent tilt changes the angle of incidence of
  the solar radiation, changing its insolation per unit area as noted in
  the diagram above.
• At the same time the tilt also changes the path length of
  the radiation through the atmosphere which in turn changes the amount of
  the Sun’s energy absorbed by the atmosphere. (also shown in the same
  diagram above).
• The tilt also changes the number of daylight hours.

These factors all work together to reduce both the intensity and daily duration of the insolation during winter months.

 

As seen from the northern hemisphere of the Earth, the
declination in the elevation of the Sun varies during the course of the
year between minus 23.45° in the summer and plus 23.45° in the winter.

Taking into account the solar declination, the altitude angle α of the sun is (90 – Θ ± δ) degrees.

The inclination angle of solar collectors from the horizontal for maximum efficiency should therefore be (Θ ± δ) degrees and the collector should be able to follow this variation in declination throughout the year.

 

• Time

Fortunately as a source of renewable energy the Sun is much
more predictable than the wind. It comes up every morning and goes down
every night. The intensity of the wind may be extremely variable, but it
is available 24 hours per day, while solar power is only available
during daylight hours. At least solar power is reliable and is
available when it is needed most – during peak demand hours.

 

Though the insolation is subject to two temporal variations, a
diurnal (daily) cycle due to the Earth’s rotation and a yearly cycle
due to the tilt of the Earth’s axis, we know precisely the magnitude of
these effects at any time so we can design our solar power systems
accordingly. What is less predictable however is the affect of the
weather.

 

Unless they are connected to the grid, systems which must
provide energy on demand need some form of energy storage or an
alternative source of energy for the hours of darkness.

 

Some Meteorology

Unfortunately we have no control over the
weather. Overcast skies can severely reduce the energy received on the
ground. Obviously solar power generating plants are best located in
regions with minimum cloud cover, dust and air pollution. At least we
usually have statistics about regional weather conditions to help in
choosing suitable locations for solar power plants.

 

For dimensioning a solar power generating
system it is essential to know the number of hours of daylight expected
at the site location. This can normally be obtained from national
meteorological services and environmental research establishments as
well as from NASA in the USA. It helps even more if they are able to
provide tables of expected solar energy for the region.

Note: It is important to check the basis of
the data. Some organisations quote the solar insolation on a horizontal
surface, that is the ground. Others base their data on the insolation of
a collector with a fixed angle of tilt corresponding to the latitude of
the location.

 

Energy Capture and Collector / Array Orientation

The table opposite shows how the effectiveness of a solar array or collector diminishes as its orientation and tilt move away from the optimum position.   The example shows that to capture maximum solar energy with an array located at a latitude of 35° North, the optimum array orientation is pointing due South and the optimum tilt is the same as the latitude, in this case 35°. If the array system has to be mounted on a roof with a pitch of 45° on a building pointing South West it will only receive a maximum of about 90% of the available solar energy.

Solar Tracking

As indicated above the amount of energy
captured by a solar system can be maximised if the collector can follow
the ecliptic path of the Sun so that the plane of the collector or array
is always perpendicular to the direction of the Sun.

Automatic mechanical tracking systems make it
possible to track both the azimuth and the elevation of the Sun’s
position to maximise energy capture.

 

 

Note the lower zenith and the reduced azimuth range of the winter Sun. The chart below shows that, in the UK, the available energy from the winter Sun is
between one sixth and one twelfth of the energy from the summer Sun
depending on the latitude.

 

• Azimuth Tracking

Azimuth tracking keeps the collector pointing at the Sun as the Earth rotates.

The insolation varies between zero and its
maximum value during the course of every day and remains around its
maximum value for a relatively short period of time. Azimuth tracking
enables the collector to follow the Sun from East to West throughout the
day and brings the most benefits.

Passive systems provide the simplest form
of azimuth tracking. They have no motors, controllers or gears and they
don’t use up any of the energy captured by the collector. They depend on
the differential heating of two interconnected tubes of gaseous
refrigerants, one on either side of the collector. If the collector is
not pointing towards the Sun, one side heats up more than the other and
vaporises its refrigerant. The resulting change in weight is used in a
mechanical drive mechanism to turn the collector towards the Sun where
it will remain when the temperature and weight of the two tubes will be
balanced.

Active tracking is also possible by
employing temperature sensors and a control system with linear actuating
motors taking their drive power from the system.

 

• Altitude/Elevation Tracking

Elevation tracking enables the collector to
follow the seasonal variations in the Sun’s altitude but the economic
benefits are less than for azimuth tracking.

Compared with the daily variations in
insolation, the seasonal variations are very slow and the range of the
variation, due to the solar declination is much more restricted. Because
of this, reasonable efficiency gains can be obtained simply by manually
adjusting the elevation of the collectors every two months. To avoid
the cost and complexity of elevation tracking, it may be more cost
effective just to specify larger collectors.

 

• Dual Axis Tracking

Combining azimuth and elevation tracking enables the
installation to capture the maximum energy using the smallest possible
collectors but the systems are complex and many installations get by
with just azimuth tracking.

 

Solar Collectors

A solar collector is simply a heat
collecting surface which intercepts the Sun’s radiated energy and heats
up a thermal working fluid. In practical thermal systems it is usually
more convenient to focus the Suns heat energy on to a small receiver in
order to obtain a higher temperature rise of the working fluid. Such
collectors are called concentrators.

Concentrators

Typical concentrators are constructed from
parabolic mirrors which reflect the Sun’s parallel rays on to a single
spot at the focus of the mirror.

• Parabolic Dish

A parabolic dish will capture the energy
intercepted by the dish and concentrate it on a suitable heat absorber
located at the focus. The amount of energy captured and hence the
temperature rise of the absorber will be proportional to the area of the
dish. Size limitations of the dish limit its application to small
systems of from 10kW to 50kW.

• Parabolic Trough

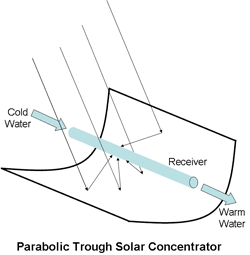
Larger systems use arrays of parabolic
trough shaped mirrors oriented north-south to concentrate the solar
radiation. They usually also include a tracking system to track the
Sun’s path throughout the day.

 



Source: US DOE (EERE)

The thermal absorber, a tube located at
along the focal line of the mirror, contains the working fluid which is
heated by the solar radiation to a high temperature and used to drive a
heat engine.

 

• Power Tower

An alternative concentrator arrangement
is the Power Tower which uses a large array of parabolic mirrors focused
on a solar furnace mounted on the top of a tower. Because of the long
focal length, the mirrors are almost flat.



 

As with the trough concentrators, the solar furnace is used to raise steam to drive a turbine generator.

 



Source: U.S. NASA

• Suns – This is a unit
  used by the solar concentrator community to express the degree of
  concentration of the mirror system, similar to the magnification factor
  of a lens. Note that this unit is not precisely defined .

 

Available Energy – Practical Systems

The table below shows the solar energy
available at two extremes of latitude and provides an indication of the
upper and lower limits of the solar energy falling on the Earth. The
insolation (kWh/m²/day) is the monthly averaged incident
energy falling on a horizontal surface at the given location. Also
called the “Equivalent Sun Hours” or “Hours of Full Sun” (See Definition)

 

Solar Energy Available at Different Latitudes
Location	Latitude Degrees	Altitude Metres	Tracking	Insolation kWh/m2/Day
				June	December
Anchorage, Alaska	61.17°N	35	None	4.5	0.6
			2 Axis	6.8	0.7
Quito, Equador	0.47°S	2851	None	4.38	4.81
			2 Axis	6.09	6.62

Source NREL

 

Because of cloud cover and pollution, the
quoted hours of “full Sun” are substantially less than the actual hours
of daylight. In sunnier climes, an average of 33% of solar irradiation
comes from diffuse light but for the majority of locations this is
typically more than 50%. The equivalent hours of full Sun takes into
account the affect of overcast or partially cloudy skies.

 

System Dimensioning – Energy Capture

Much care is needed in specifying solar array
sizes to meet system power requirements. Using yearly average
insolation figures for the chosen location may be acceptable if all that
is required is a grid connected system with an average annual
generating capacity, but this is almost never the case and it certainly
does not apply to stand alone systems.

Averages can be very misleading, even within the month.

The following table gives the monthly average, and yearly average, insolation at two locations in the UK.

 

Daily Insolation Levels (kWh/m2/day) at Locations in the UK
Location	Latitude	Longitude	Jan	Feb	Mar	Apr	May	June	July	Aug	Sept	Oct	Nov	Dec	Average
Edinburgh	55′ 55″ N	3″ 10″ W	0.44	0.94	1.86	3.18	4.33	4.34	4.13	3.41	2.43	1.2	0.59	0.32	2.26
London	51′ 32″ N	0′ 5″ W	0.67	1.26	2.22	3.48	4.54	4.51	4.74	4.01	2.86	1.65	0.89	0.52	2.61

Monthly Averaged Insolation Incident On A Horizontal Surface (kWh/m²/day)

Source NASA

 

If the system capacity were to be based on
the yearly average, for most practical installations there would be a
surplus of energy in the summer and a shortfall in winter. A stand alone
system would have to be dimensioned to be able to provide the peak load
during the winter months, otherwise an auxiliary source of power must
be provided. The system would then be over-specified for the summer
months and some form of reducing the capacity or dumping the excess
energy must be found. A hybrid system combining wind and solar power could be the answer.

 

Energy Storage

Because no power is provided during the hours
of darkness, the stand alone systems must generate and store sufficient
energy during the day to satisfy the peak daily load. The storage
should also be sufficient to cover several days when no sunlight is
available. Batteries are normally used as a buffer to provide the
necessary storage to guarantee short term continuity of supply by
storing surplus energy during the day for use during the night and
during periods of overcast skies. Unfortunately it is not practical to
store the summer’s surplus energy for use during the winter.

 

Solar Power Generation (Thermal)

Electricity generation in a solar thermal
plant occurs in two stages. First the heat energy from the Sun is
captured and used to heat a working fluid which is then used in a second
energy transformation stage to generate the electricity. Note that the
thermal energy comes from the Sun’s radiation and not from the air whose
temperature will usually be much lower than the temperature of the
working fluid. The actual operating temperature reached by the working
fluid will depend on the rate at which the thermal energy is being
extracted by the working fluid (the flow rate) and delivered to the
electricity generating system.

A solar thermal power plant usually has a
system of mirrors to concentrate the sunlight on to an absorber, the
absorbed energy then being used to power a heat engine which in turn
drives a rotary generator. In large scale systems, the heat engine is
usually a turbine driven by steam or other vaporous working fluid. In
small scale systems the heat engine may be a Stirling engine.

 

Electricity Generating Systems

Large Scale Thermal Plants

The system below is designed to capture the thermal energy radiated from the sun.

 

 

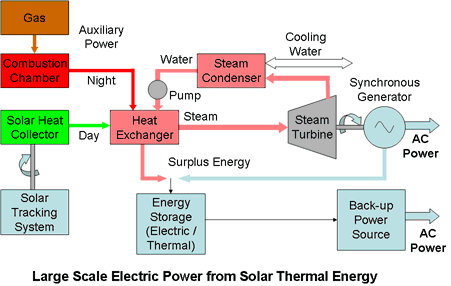
Thermal energy from the Sun is intercepted by
a concentrator which focuses the energy on a heat absorber containing
the working fluid, usually a synthetic oil, which is heated by the solar
radiation to a high temperature typically 400° C. The system may use a binary cycle in which the heated oil is passed through a heat exchanger to raise
steam which is used to drive a conventional turbine and generator in a
separate circuit.

To maintain the thermal efficiency of the
turbine, the working fluid leaving the heat exchanger should not be
allowed to cool down. Solar plants are therefore supplemented by
gas-fired boilers which generate about a quarter of the overall power
output and maintain the temperature overnight.

Several such installations in modules of 80
MW are now operating and solar conversion efficiencies of between 15%
and 23% have been achieved. Each module requires about 50 hectares of
land and needs very precise engineering and control. Power costs are two
to three times that of conventional sources.

 

Small Scale Thermal Plants

Steam turbines are only practical for very large installations. Stirling Engines are often used in small systems to drive the electrical generator.

 

Solar Stirling

Domestic thermal generating
plants typically use an array of water filled panels or a small array of
parabolic trough concentrators to capture the Sun’s thermal energy.
Very small system such as those used in space applications may simply
use a parabolic dish to capture the energy.

 

 

The working fluid is then used as the
external heat source for powering the Stirling engine which in turn
drives a rotary generator.

An off-grid stand alone solar electric system must have batteries supported by Balance-of-System (BOS) components including chargers, inverters and controllers to manage the
energy flows in order to provide power on demand. This makes the system
very expensive. Grid connected systems also need power conditioners and
control systems if surplus energy is to be sold back to the utility
company.

Efficiencies achieved with small scale systems range from 18% to 23%.

 

Domestic Water Heating Applications – A brief diversion

Many small domestic solar thermal systems are merely used for water heating and not for generating electricity.

 

• Practical Systems

The working fluid is water, circulating
through a rooftop mounted solar panel and fed directly into the
domestic hot water system. As an alternative, the working fluid may be
passed through a heat exchanger consisting of a coiled pipe in the hot
water storage tank to heat the water indirectly.

The front surface of the solar panel is
double glazed, allowing the Sun’s radiation to pass through to heat up
the water flowing through the panel while preventing heat loss from the
warmer water due to convection and conduction in the opposite direction
(from the panel to the colder atmosphere). The rear surface of the panel
is also insulated to prevent heat loss in that direction.

The system works in cold weather because
the water is heated by the Sun’s radiation, not by the ambient air from
which it is insulated.

 

• Water Temperature

An elegant, self regulating solution for
maintaining the water temperature is provided by incorporating a small,
subsidiary photovoltaic panel (see below) to generate the electrical energy needed to power the water circulation pumps instead of using mains electricity.

At sunrise, the pump remains switched off
until the water reaches its operating temperature at which point the
pump is switched on. As the Sun’s radiation increases during the
morning, the water temperature will rise, but at the same time the solar
powered pump will run faster, increasing the water flow and thus
transfering heat more quickly from the panel to the hot water storage
tank. By suitably dimensioning the pump and the photovoltaic panel, the
heat transfer rate from the panel can be matched to the heat absorption
rate from the Sun thus maintaining a constant water temperature. As the
received Sun’s energy wanes in the afternoon the process is reversed,
the pump runs more slowly reducing the rate at which heat is extracted
from the panel thus maintaining its temperature. Being completely
independent of the electricity grid, these systems have the added
economic and environmental benefits that no electrical energy is drawn
from the grid for running the pumps.

• Temperature Limits

With water as the working fluid, the system
is prone to freezing and boiling unless special precautions are taken.
Low cost systems allow the water to freeze in very cold and dark
environments by using flexible freeze-tolerant, silicone rubber
pipework which is sufficient to accommodate the expansion of the water
as it turns to ice. The volume of water used in solar thermal panels is
very small, typically around 2 or 3 litres and is spread over a very
large area to capture the maximum solar radiation. The high received
radiation acting on a low water volume enables the water to heat up very
quickly but for the same reason makes it susceptible to boiling. Unless
there is a constant water flow to a storage tank with the heated water
in the panel being replaced by cold water from the tank, the water could
reach temperatures of 150 degrees C or more and for this reason the
water pumps must be continually switched on. Even so, the possibility of
boiling still remains, even with the pumps running, if the system is
incorrectly dimensioned. The equilibrium temperature reached will depend
on the balance between the solar energy captured by the panel and the
thermal energy absorbed in the storage tank, the rate at which it is
withdrawn from the tank and the system heat losses. Using a very small
panel coupled to a very large tank with high hot water usage will result
in a low water temperature in the tank. Conversely using a very large
panel with a very small tank could result in boiling, particulaly if
the hot water usage is very low. This need not be a disaster since the
water content in the panel is very low and system could be designed to
allow the steam to vent in case of boiling.

• Efficiency

Energy conversion efficiencies achieved in
these pure thermal applications may be three or four times the
efficiency of photovoltaic applications though their applications are
much more limited.

• Economics

In higher latitudes the available solar
energy captured by practical domestic installations may be sufficient to
provide hot water for washing and showering but not enough to supply
building space heating requirements during the colder months. Back-up
heating systems will consequently be needed to cater for the base load
to satisfy these requirements. Because the supply of solar energy is
intermittent, the conventional heating system must fill in the gaps and
there is little opportunity to downsize it. The householder will
therefore, most likely have to pay the capital costs of a base load
system capable of supplying the full heating load as well as the solar
heating system even though the conventional heating system will not be
working at full capacity most of the time.

Domestic solar thermal systems may not
generate electricity directly but they do contribute to a reduction in
the use of electrical energy and its associated costs.

• Example

Useful Energy Captured

The table above shows that in the UK, the average solar radiation received is about 2.5 kWh / M² / day. A single solar panel with an area of 3 M² will therefore capture 2.5 x 3 x 365 = 2737 kWh of energy per year.
With a system conversion efficiency of around 40% and less than optimal
orientation of a typical rooftop mounted solar panel, the maximum usable
energy received by a single panel system will be around 1000 kWh. This
is roughly equivalent to the energy supplied by a 3 kW immersion heater
used for one hour per day. As always however, averages can be
misleading. In the summer, the solar panel could deliver an “average”
of about 5 kWh of heating energy per day, but in the winter this could
be as low as 0.4 kWh per day. The energy captured can of course always
be increased by increasing the number of solar panels employed in the
system.

Cost Savings

The cost saving will depend on whether the
solar system is replacing 1000 kWh of heating energy supplied by a gas
or an electric water heating system and the associated tarriff charged
for the energy. With UK domestic gas currently costing less than £0.03
per kWh ($0. 045) and electricity costing about £0.10 per kWh ($0.15)
the annual savings are likely to be somehwere between £30 and £100 ($50
to $150).

Since typical single panel installations
cost around £2,500 or £3,000 ($4,000 to $5,000), unless the systems
qualify for a government subsidy or there is a very large increase in
energy costs, the payback time for the investment will be measured in
decades rather than years. Saving the planet can be quite expensive.

Carbon Footprints

As with wind power, if the investment fails the conventional economic tests, the notion of carbon footprints is often used to jusify the expense, based on the potential for
reducing the amount of greenhouse gases emitted by alternative methods
of power generation.

 

See also Domestic Solar PV System Economics below.

 

Solar Power Generation (Voltaic)

Solar voltaic power generation is the direct conversion of solar energy into electricity.

Sunlight comes in many colours, combining low-energy (1.1 electronVolts (eV)) infrared photons with high-energy (3.5 eV) ultraviolet photons and all the rainbow of
visible-light photons in between. Solar cells, also called photovoltaic
or PV cells, are semiconductor devices designed to capture these photons
and convert their energy directly into electrical energy.

 

How Solar Cells Work

When a photon with sufficient energy impinges
upon a semiconductor it can transfer enough energy to a electron to
free it from the bonds of the semiconductor’s valence band so that it is
free to move and thus carry an electric current. The junction in a
semiconductor diode provides the necessary electric field to cause the
current to flow in an external circuit.

A more detailed explanation of how solar cells work is given in the section on photovoltaic diodes.

 

The typical output voltage of a PV cell is
between 0.5 and 0.6 Volts and the energy conversion efficiency ranges
from less than 10% to over 20%. An array of cells can therefore
generate about 200 Watts of electrical power per square metre when
illuminated by solar radiation of 1000 Watts per square metre. The
corresponding current density will be about 400 Amps/m². Because of climatic conditions the intensity of the insolation rarely reaches 1000 W/m².

Practical cells are also much smaller than
one square meter with actual sizes of commercially available cells
ranging from about one centimetre square to 15 centimetres square. The
corresponding output Wattages for these cells range from 20 milliWatts
to about 4 Watts.

 

PV Cell and Module Ratings

• Standard Test Conditions (STC)

In order to compare solar cells on a like
for like basis a set of Standard Test Conditions (STC) has been defined.
The conditions are: normal irradiance of 1000w/m² , cell temperature 25 °C and Air Mass =1.5

• Air Mass

The receiving surface corresponding to AM 1.5 is defined as
an inclined plane at 37° tilt (the average latitude in the USA) toward
the equator, facing the sun. In this case, the surface normal points to
the sun, at an elevation of 48.81°, its zenith angle, above the horizon.

• Rated Power

Rated Power is defined as the maximum power (Wp or kWp) generated by the cell or module under the Standard Test Conditions.

 

Solar Cell Operating Characteristics

The graph below shows that with constant
irradiance the output voltage of a cell or an array of cells falls as it
is called upon to deliver more current.

 

Maximum power delivery occurs the voltage has dropped to about 80% of open circuit voltage voltage.

The Fill Factor (FF) is defined as the ratio
between the power at the maximum power point and the product of the open
circuit voltage and short circuit current. It is typically better than
75% for good quality solar cells.

 

The short circuit (SC) current is directly related to the number of photons absorbed by the semiconducting material and is thus proportional to light intensity. The conversion efficiency is therefore reasonably constant so that the power output is proportional to the irradiance down to fairly low levels, however the efficiency is reduced if the cell temperature is allowed to rise. The open circuit (OC) voltage varies only slightly with light intensity.

As temperature increases, the band gap of the intrinsic semiconductor shrinks, and the open circuit voltage (Voc) decreases. At the same time, the lower band gap allows more incident energy to be absorbed because a greater percentage of the incident light has enough energy to raise charge carriers from the valence band to the conduction band. A larger photocurrent results. The increase in the current for a given temperature rise however is proportionately lower than the decrease in voltage. Hence the efficiency of the cell is reduced.

 

Solar Cell Efficiency

The following graphs show the same information as those above
but in a slightly different form showing how increased temperature
reduces the efficiency.

 

 

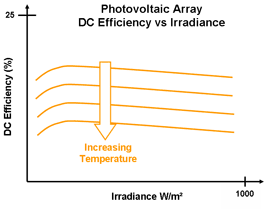
In real outdoor conditions the rated peak
power Wp is seldom achieved, since module temperature usually is more in
the range of 40°C – 60°C. Efficiency can be improved by cooling the
cells and some systems have been designed to make use of the heat
absorbed by the cooling fluid in solar heating applications.

 

Solar Cell Types

Several types of solar cells have been developed with the aims of reducing costs and improving efficiencies.

• Crystalline Silicon Solar Cells

Benefiting from the manufacturing experience of the
semiconductor industry, crystalline silicon is the leading solar cell
material, though still relatively expensive. Monocrystalline cells are
cut from single crystals of high purity electronics grade silicon.
These cells are about 25 percent efficient at best. Using the easier to
manufacture polycrystalline silicon cut from from a block of crystals
or less pure, so called “solar grade” silicon, efficiencies may be only
about 15% or 16% due to the effect of grain boundaries or impurities but
they cost a fraction of single crystal electronics grade cells.

• Amorphous Silicon Solar Cells

Amorphous Silicon has been employed for many years in the
manufacture the solar cells used for powering electronic calculators and
watches and promises the possibility of low cost, higher power cells.
Amorphous material appears like a solid but has no regular crystal
lattice structure. Glass is an example of such materials. The presence
of controlled quantities of certain “impurity” elements such as hydrogen
and the random crystal lattice formation actually enhance the otherwise
very low conversion efficiency. Typical cell efficiencies range from 5%
to 10%.

Manufacturing yield is still a problem and the cells suffer from degradation when exposed to the sun.

• Thin Film Silicon Solar Cells

Thin film cells are made by depositing the active photovoltaic
material, such as amorphous silicon or other semiconductor onto a glass
or other substrate together with the necessary current collecting
contacts. The cell construction is much less costly than using
semiconductor wafers and the manufacturing process is also simpler as
well as being suitable for making cells with a much larger area and
hence current carrying capability. Efficiencies of 11% to 14% have been
achieved with this construction.

PV systems on flexible polymer substrates have also been made
using Copper Indium Gallium Selenide (CIGS) active material with
efficiencies of 10%.

• Multi Layer (Tandem) Solar Cells

Better conversion efficiencies are possible by using multiple
layers of differing semiconductor materials, optimised for different
wavelengths, in a single device. This can raise the theoretical
efficiency limit, currently about 30% for a single junction device, to
about 45% for a three junction cell.

Efficiencies of over 33% have already been achieved in practical devices.

• Exotic Materials

Materials such as Gallium Arsenide, Copper Indium Diselenide,
Cadmium Telluride and Indium Nitride have been employed to provide
particular characteristics to optimise solar cells for specific
applications.

Gallium Arsenide is used for military and aerospace
applications in a variety of cells in combination with other elements
because of it’s suitability for capturing high energy photons (ultra
violet radiation), high potential conversion efficiency and its ability
to withstand high temperatures. It is however more difficult to
manufacture and cells using Gallium Arsenide can be 100 times more
expensive than commercial silicon based cells.

Copper Indium Diselenide and Cadmium Telluride are used in
polycrystalline form in low cost thin film cells because of their ease
of manufacture and reasonable yields. Efficiencies are however low
ranging from 8% to 14%

Indium Nitride is suitable for capturing low energy photons
(infra red radiation) making it suitable for full spectrum devices when
used in tandem solar cells in combination with other materials such as
Gallium Arsenide which capture the high energy photons.

• Electrochemical Solar Cells – Dye Sensitised Solar Cells (DSSC or Grätzel Cells)

Relatively new, these cells are low cost devices which use dye
sensitised Titanium dioxide in combination with a liquid electrolyte
to generate the current. Up to now they are only available in small
sizes with efficiencies between 7% and 10%.

 

Solar PV Collectors

Solar cells are usually sold in modules built
up from a number of cells arranged in series and / or parallel to
provide convenient or commonly used voltages and power ratings.

Solar Arrays

Modules can be similarly interconnected to create larger arrays with the desired peak DC voltage and current.

 

Concentrators

As with thermal collectors, concentration of
the incident energy on to a smaller surface is possible. For very small
applications, optical mirrors and lenses are used.

 

Maximum Power Point Tracking (MPPT)

A power source will deliver its maximum power
to a load when the load has the same impedance as the internal
impedance of the power source. (Jacobi’s Law). Unfortunately, batteries are far from the ideal load for a solar array and the mismatch results in major efficiency losses.

A typical PV array designed to charge 12 Volt
batteries delivers its maximum power at an operating voltage around 17
Volts. Lead Acid batteries are normally charged up to 14 Volts though
the voltage quickly drops to 12 Volts as they start to deliver current
and lower still as the depth of discharge (DOD) increases.
In its simplest form, charging is carried out by connecting the PV array
directly across the battery. The battery however is a power source
itself and presents an opposing voltage to the PV array. This pulls the
operating voltage of the array down to the voltage of the discharged
battery and this is far from the optimum operating point of the array.

The diagram below shows the performance of a17
Volt, 4.4 Amp, 75 Watt PV array used to top up a 12 Volt battery. If
the actual battery voltage is 12 Volts, the resulting current will only
be about 2.5 Amps and the power delivered by the array will be just over
50 Watts rather than the specified 75 Watts: an efficiency loss of over
30%.
Maximum Power Point Tracking is designed to overcome this problem.

 

 

The power tracker module is a form of voltage regulator
which is placed between the PV array and the battery. It presents an
ideal load to the PV array allowing it to operate at its optimum
voltage, in this case 17 Volts, delivering its full 75 Watts regardless
of the battery voltage. A variable DC/DC converter in the module
automatically adjusts the DC output from the module to match the battery
voltage of 12 Volts.
As the voltage is stepped down in the DC/DC converter, the
current will be stepped up in the same ratio. Thus the charging current
will be 17/12 X 4.4 = 6.2 Amps and, assuming no losses in the module,
the power delivered to the battery will be 12 X 6.23 = the full 75 Watts
generated by the PV array.
In practice the converter losses could be as high as 10%.
Nevertheless a substantial efficiency improvement is possible.

 

It is not enough however to match the
voltage at the specified maximum power point (MPP) of the PV array to
the varying battery voltage as the battery charges up. Due to changes in
the intensity of the radiation falling on the array during the day as
well as to changes in the ambient temperature, the operating
characteristic of the PV array is constantly changing and with it the
MPP of the PV also changes. Thus we have a moving reference point and a
moving target. For optimum power transfer, the system needs to track the
MPP as the solar intensity and ambient temperature changes in order to
provide a dynamic reference point to the voltage regulator.

High performance MPPT modules may
incorporate software algorithms to take account of the variations in
insolation and temperature. A typical job for fuzzy logic or a neural network. Alternatively the optimisation can be accomplished in hardware by means of a perturbation signal incorporated in a feedback loop which drives the system operating point to the MPP.

A small dither voltage is
superimposed on the PV voltage and its affect on the regulator output
current feeding the battery is monitored. If the current drawn by the
battery increases when the dither voltage increases, then the operating
point has moved towards the MPP and therefore, the operating voltage
must be increased in the same direction. On the other hand, if the
current into the battery decreases, then the operating point has moved
away from the MPP and the the operating voltage must be decreased to
bring it back.

 

Large Scale Photovoltaic Plants

Several large scale grid connected PV power plants have been
constructed throughout the world, mostly of 300 kW to 500 kW capacity
but some as high as 10MW. Up to now deployment of large scale plants has
been limited to experimental installations by the high cost of the
solar panels. With typical efficiencies of 10% or less, a 500 kW plant
will need over 5000 square metres of PV panels costing $1.0 per Watt as
well as large scale inverters capable of handling the full system power
output.

 

Small Scale Photovoltaic Plants and Domestic Applications

The diagram below shows the basic building blocks of a small
stand-alone off-grid PV power generating system. A grid connected
system would not need the battery and MPPT power tracking system. They
do however need alternative capacity to come on stream to carry the load
during the hours of darkness.

 

 

Photovoltaic System Dimensioning

 

• Array sizes for Photovoltaic System

The following example show the array sizes necessary to
generate 10 kWh of usable energy with an average daily insolation of 2
kWh/m²/day. Note that the results are heavily dependent on the efficiency assumptions used.

Needless to say the array must not be shaded by objects such as trees or buildings.

 

• Example

Energy received per unit area = Insolation X Solar conversion efficiency.

Thus:

The area required for a given energy capture = Energy required ÷ ( Insolation at the desired location X Solar conversion efficiency)

Using an efficient (expensive) photovoltaic array with a conversion efficiency of 15% the area of the array will be:

10÷(2 X 0.15) = 33.3 m²

Insolation data is usually provided for the energy falling
on a flat surface. By tilting the array to an angle corresponding to the
latitude of the location, an extra 10% of energy can be captured
reducing the area required to 30 m². See the diagram showing Array Orientation

This advantage will be lost however if the array is to be mounted on a roof which is not optimally aligned towards the Sun.

If the array is free standing on the ground, and not
constrained to be used on a roof, a solar tracking system can be used
to enable more of the Sun’s energy to be captured. A 30% improvement is
possible reducing the required array area to about 21 m²

 

Note that the PV array output is DC electrical power.

To provide AC power there would be further electrical losses
of 10% to 20% in the voltage regulator, inverter and control circuits.

 

• Grid-connected Systems

Assuming 20 % electrical system losses, a fixed PV array with an area of around 36 m², or a solar tracking PV array of 25 m² would be required to provide 10 kWh of AC power per day.

 

• Stand Alone Systems

Off-grid systems are subject to the same performance
parameters as grid-connected systems however since they also use battery
storage they suffer from an extra efficiency loss of up to 30% due to
the back emf of the battery.

Unless an MPPT tracking system is used to reduce these losses the array would have to be 30%
bigger to compensate. Thus to provide the same 10 kWh of AC power per
day in a stand-alone system, the required PV array area would have to be
47 m² for a fixed installation and 33 m² for a solar tracking system.

 

Electricity consumption in many households in Europe and the
USA is 2 or 3 times more than 10 kWh per day, particularly for those
willing to invest in solar PV electricity generation. (See Energy Demand Table). This implies that very large PV arrays with areas up to 150 m² or more, probably larger than the available South facing roof surface, would be needed to satisfy their energy demands.

 

All of the above is based on an average insolation of 2 kWh/m²/day,
but in northern temperate zones the winter insolation is likely to be
less than a quarter of the average for the location. See the table for Energy Availability and Energy Capture above. Thus the available energy will be only 2.5 kWh/day during the
winter months or the systems would need to be four times bigger in order
to supply the same 10 kWh/day of electrical energy in the winter.

 

Domestic Solar PV System Economics

Example

• Costs

According to the UK Government Energy Saving Trust, the
costs for installing a solar PV system vary greatly. An average
domestic system is quite small generating around 2.2 kWp and costs
around �12,000 ($18,000). Larger solar electricity systems can cost in
the region of �4,500 ($7,000) to �8,000 ($12,000) per kWp, reducing
slightly as the system size increases.

• Benefits

A 2.2 kWp system only delivers the full 2.2 kW of power under Standard Test Conditions of 1000 W/m² insolation. It would generate 52.8 kWH (52.8 Units) of electricity per
day if the Sun was directly overhead and shining constantly day and
night. But the table above shows that the average insolation in the UK is only about 2.5 kWh/m²/day. This is equivalent to 2.5 hours of full Sun (see EHS above) per day, not 24 hours. Thus the actual electrical energy output
from the PV system in the UK will be about 5.5 kWh per day or 2,000 kWh
per year.

• Payback

Buying 2,000 kWh of electrical energy from the local utility
company would cost £200 ($300) with the curent costs of electricity at
£0.10 ($0.15) per unit. Ignoring maintenance costs, this gives a payback
period of sixty years.

Fortunately, many governments provide generous grants to
subsidise the installation and/or operation of solar power systems thus
reducing the capital outlay and decreasing the investment payback time.

•  Selling surplus energy back to the utility company

The average UK household consumes about 5,000 kWh of
electrical energy per year or around 14 units per day. The likelihood of
a domestic installation as described above having regular surpluses is
quite remote.

Furthermore, feeding electrical energy back into the grid
involves the obligatory installation of additional, costly metering and
safety systems as well as synchronisation electronics so that this
option is only economically justifiable for installations with
relatively large surplusses.

 

Beware when the solar panel salesman comes knocking!

 

See also Domestic Solar Heating and a comparison of Electricity Generating Costs for different fuels.

 

• Balance of System (BOS) Components

The associated BOS components needed to complete the system are described in the section on Small Scale Systems.

 

• Other PV System Considerations

Converting the direct current output of the
PV array to alternating current is both costly and inefficient. Some of
this cost and waste can be avoided by using household appliances
designed to run on DC power where they are available.

Similarly it is not sensible to run heaters from PV systems.